
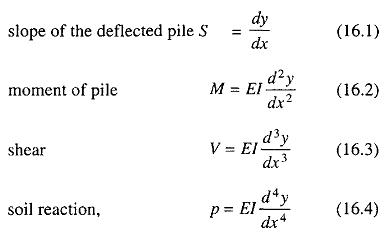
We will learn in detail about the procedure of application of “Double integration method” to find slope and deflection in beams in following numerical problems. In equations (9) and (10) the values of integration constants ( C'_1 \ \& \ C'_2 ) are obtained from given end conditions of beam. Equation (10) gives deflection at any section of a beam.Haptic, flexible amplification mechanism, amplification ratio, flexure hinges. M = EI \left ( \frac \right ) \iint M - C'_2 ………… (10) The amplification ratio of formula calculation and FEA (Finite Element. It is the main point of consideration by the engineers and designers while designing of a beam.įrom differential equation of flexure, we have got the relation – Importance of Flexural Rigidityįlexural rigidity determines the strength of a beam. Therefore, flexural rigidity of a beam is defined as the product of modulus of elasticity for the material of beam and moment of inertia for the section of the beam. Flexural stiffness is the measure of ability of a member undergoing deformation It gives the resistance offered by a structural member while undergoing bending moment. Flexural Rigidity is the term which determines the strength of any structural member.įlexural rigidity is defined as the bending moment required to produce unit curvature in a beam. They can be stretched, compressed and bent. Solid bodies are said to be rigid but, in reality they are not perfectly rigid. A body having good rigidity will resist any type of deformation under the influence of load. Rigidity is the property of any material by virtue of which it offers resistance to deformation due to an applied load.


 0 kommentar(er)
0 kommentar(er)
